Adding and subtracting fractions with unlike denominators can feel tricky at first. But don’t worry, I’m here to walk you through a simple way to help you add and subtract fractions with unlike denominators.
Let’s jump into this step-by-step guide with examples.
Adding Fractions with Unlike Denominators
When fractions have different denominators, you can’t just add the fractions as they are. First, you need to find a common denominator. This is a number that both denominators can be divided into evenly.
Here’s how:
Step 1: Find a Common Denominator
Look for the least common denominator (LCD). It is the smallest number both denominators can fit into.
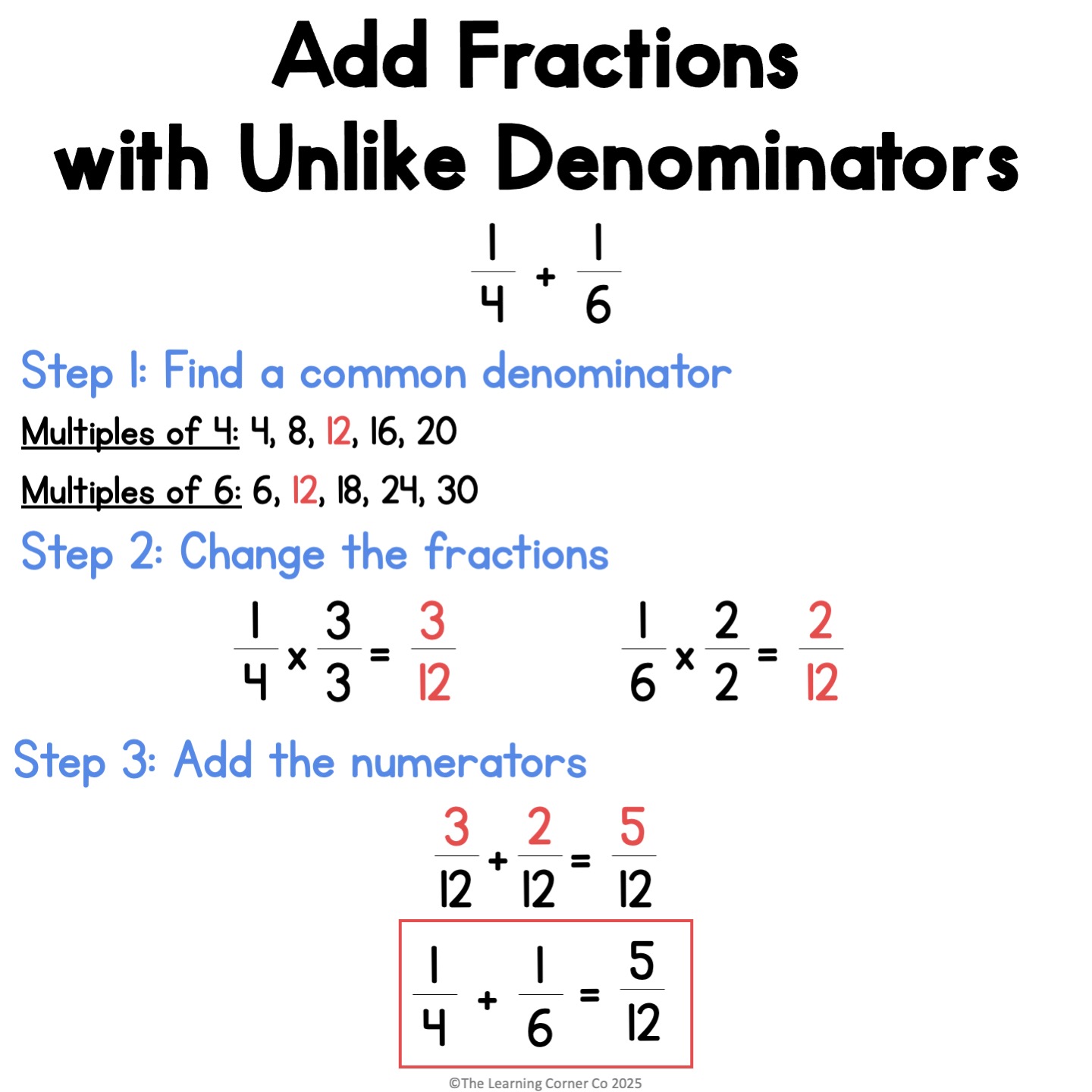
Example: Add 1/4 + 1/6.
- The denominators are 4 and 6.
- List the first five multiples of 4 and 6.
- Multiples of 4: 4, 8, 12, 16, 20
- Multiples of 6: 6, 12, 18, 24, 30
- The common multiple is 12.
Now you know 12 is your least common denominator.
Step 2: Change the Fractions
Rewrite each fraction so they both have 12 as the denominator.
- For 1/4: Think, “What times 4 equals 12?” (Answer: 3)
- Multiply the top (numerator) and bottom (denominator) numbers by 3.
1 × 3 = 3, and 4 × 3 = 12 → So, 1/4 becomes 3/12. - For 1/6: Ask, “What times 6 equals 12?” (Answer: 2)
- Multiply the numerator and denominator by 2.
1 × 2 = 2, and 6 × 2 = 12 → So, 1/6 becomes 2/12.
Step 3: Add the Numerators
Now that the fractions have the same denominators, simply add the numerators and keep the denominator same.
3/12 + 2/12 = 5/12
So, ¼ + ⅙ = 5/12.
Subtracting Fractions with Unlike Denominators
Now, let’s try subtracting fractions with unlike denominators. Subtracting works the same way as adding fractions: find the least common denominator first.
Step 1: Find a Common Denominator
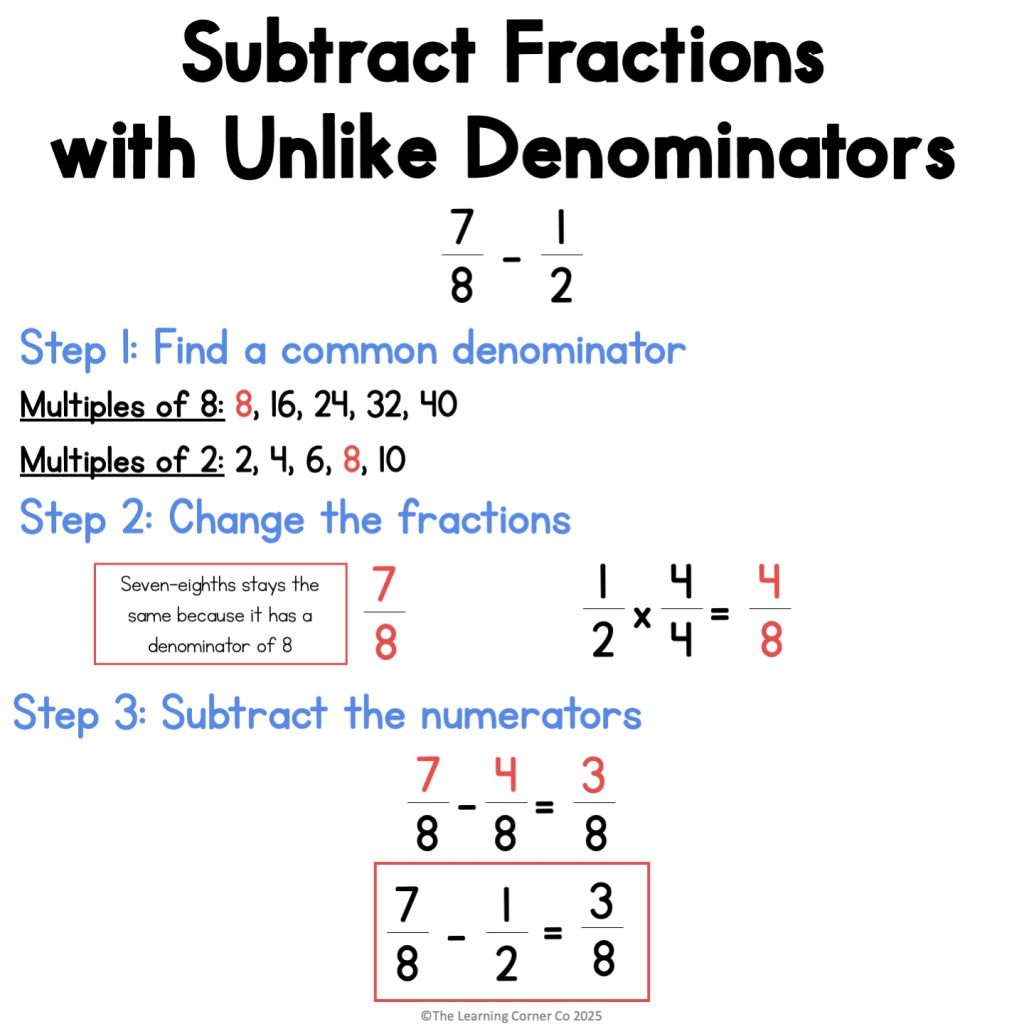
Example: Subtract ⅞ – ½.
- The denominators are 8 and 2.
- List the first five multiples of 8 and 2.
- Multiples of 8: 8, 16, 24, 32, 40
- Multiples of 2: 2, 4, 6, 8, 10
- The common multiple is 8.
Now you know that the least common denominator is 8.
Step 2: Change the Fractions
- For ⅞: Since it already has 8 as the denominator, there is no need to change the fraction.
- For ½: Think, “What number times 2 equals 8?” (Answer: 4)
- Multiply the numerator and denominator by 4.
- 1 x 4 = 4 and 2 x 4 = 8 So, ½ becomes 4/8.
Step 3: Subtract the Numerators
Now subtract the numerators.
⅞ – 4/8 = 3/8
So, ⅞ – ½ = ⅜.
Reduce Fractions to Their Simplest Form
Whenever you add or subtract fractions, it is important to check if your answer can be simplified.
Let’s look at this example.
Example: Add 10/12 + ⅓.
Step 1: Find the common denominator by listing multiples.
- Multiples of 12: 12, 24, 36, 48, 60
- Multiples of 3: 3, 6, 9, 12, 15
- The least common denominator is 12.
Step 2: Change the fractions.
- For 10/12 stays the same since it has a denominator of 12.
- For ⅓: Multiply the numerator and denominator by 4.
- 1 x 4 = 4
- 3 x 4 = 12
- So, ⅓ = 4/12
Step 4: Add the fractions.
- Add the numerators. 10 + 4 = 14
- So, 10/12 + 4/12 = 14/12
Step 5: Write the fraction in simplest form.
- 14/12 is an improper fraction because the numerator is bigger than the denominator.
- Divide 14 ÷ 12 = 1 remainder 2.
- Rewrite it as a mixed number: 1 and 2/12
- Then, simplify 2/12 by dividing the numerator and denominator by 2.
- Divide the numerator and the denominator by 2.
- 2 ÷ 2 = 1
- 12 ÷ 2 = 6
- So, 2/12 simplifies to ⅙.
So, 10/12 + ⅓ = 1 ⅙.
Tips for Adding and Subtracting Fractions with Unlike Denominators
- Use visuals: Draw circles or bars to show fractions. Kids often understand faster when they can see the math!
- Practice Finding the LCD First: Turn it into a fun matching game before adding or subtracting.
- Always Simplify: Once they’ve added or subtracted, double-check if the answer can be simplified.
Want Some Extra Help?
If you want more ready-to-go practice, I’ve created easy-to-use worksheets for adding and subtracting fractions with unlike denominators. They are perfect for reinforcing these step-by-step reminders, and lots of space for kids to work through problems.
Click on the images to check out the worksheets.
And if your child could use even more support, check out my Add and Subtract Fractions Workbook. It’s filled with lessons, review, and practice pages to help master this skill.