Adding and subtracting mixed numbers can feel confusing at first. After all, you’re working with both whole numbers and fractions at the same time! But don’t worry – once you break it down step by step, it becomes much easier.
In this guide, you’ll learn exactly how to add and subtract mixed numbers, whether the fractions have the same denominator or different ones, and what to do when subtraction requires borrowing (renaming).
Adding and Subtracting Mixed Numbers with the Same Denominator
When the fractions already have the same denominator, adding and subtracting mixed numbers is easy.
Step 1: Add or Subtract the Fractions
Start with the fractions. Add or subtract the numerators (the numbers on top) and keep the denominator (the number on the bottom) the same.
Step 2: Add or Subtract the Whole Numbers
Next, add or subtract the whole numbers.
Step 3: Simplify if Needed
If the fraction is improper (where the numerator is bigger than the denominator), change it into a mixed number.
Example: Add 3 ⅖ + 4 ⅕
- Check that the denominators are the same.
- Add the fractions by adding the numerators. Keep the denominator the same. 2/5 + 1/5 = 3/5
- Add the whole numbers: 3 + 4 = 7
So, 3 ⅖ + 4 ⅕ = 7 ⅗
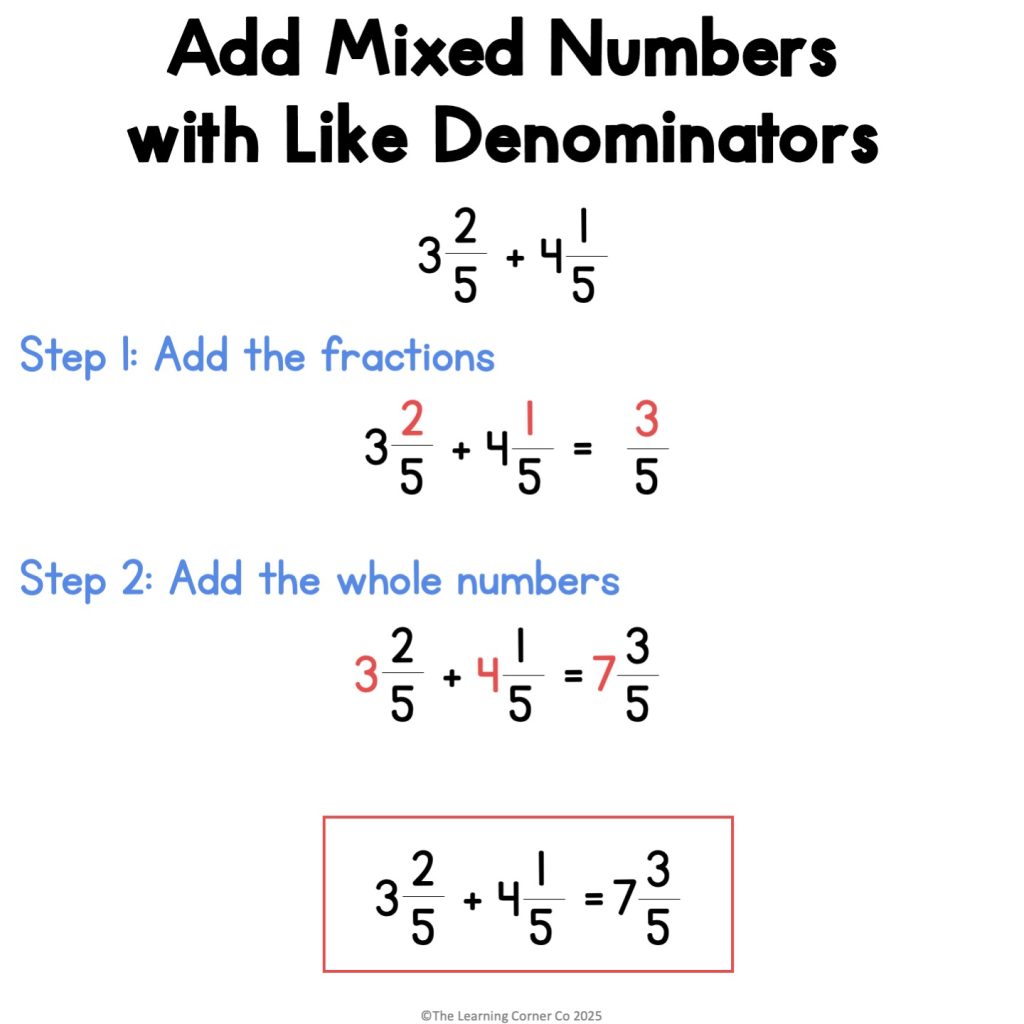
Example: Subtract 6 4/7 – 2 1/7
- Check that the denominators are the same.
- Subtract the fractions by subtracting the numerators. Keep the denominators the same. 4/7 – 1/7 = 3/7
- Subtract the whole numbers: 6 – 2 = 4
So, 6 4/7 – 2 1/7 = 4 3/7
Adding Mixed Numbers with Different Denominators
When the mixed numbers have unlike denominators (different denominators), you’ll need to find a common denominator before adding the mixed numbers.
Step 1: Find the Least Common Denominator (LCD)
Look for the smallest number that both denominators divide into evenly. You can do this by finding the common multiple of the denominators or by multiplying the denominators.
Step 2: Rewrite the Fractions
Change the fractions so that they have the same denominator.
Step 3: Add Fractions and Whole Numbers
Add the fractions first. Then add the whole numbers.
Step 4: Simplify
If your fraction is improper, turn it into a mixed number. If your fractions is not in simplest form, write it in simplest form.
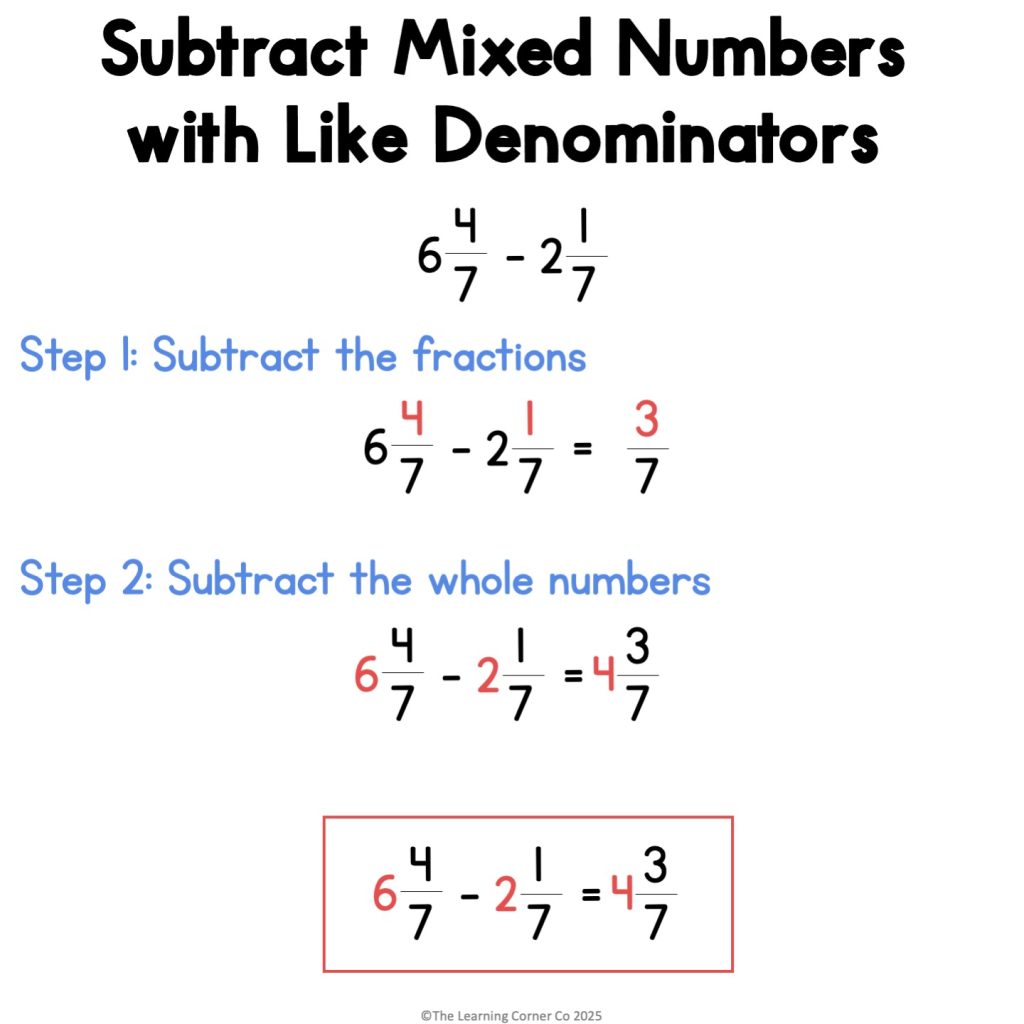
Example: Add 2 ⅓ + 3 ¾
- Find the LCD of 3 and 4 → 12
- Rewrite:
- 1/3 = 4/12
- 3/4 = 9/12
- Add the fractions: 4/12 + 9/12 = 13/12
- Since 13/12 is an improper fraction, write it as a mixed number. 13/12 = 1 1/12
- Add the whole numbers: 2 + 3 = 5
- Add the extra 1: 5 + 1 = 6
So, 2 ⅓ + 3 ¾ = 6 1/12
Need more practice like this?
My Adding and Subtracting Mixed Numbers Worksheets are packed with examples just like this one — easy to follow and great for extra confidence!
Subtracting Mixed Numbers with Different Denominators
Subtracting mixed numbers with different denominators follows the same first steps: find a common denominator before subtracting.
Step 1: Find the Least Common Denominator
Look for a common multiple or multiply the denominators to find the LCD.
Step 2: Rewrite the Fractions
Change each fraction to have the same denominator.
Step 3: Subtract Fractions and Whole Numbers
Subtract the fractions first, then the whole numbers.
Step 4: Simplify
Simplify your final answer if possible.
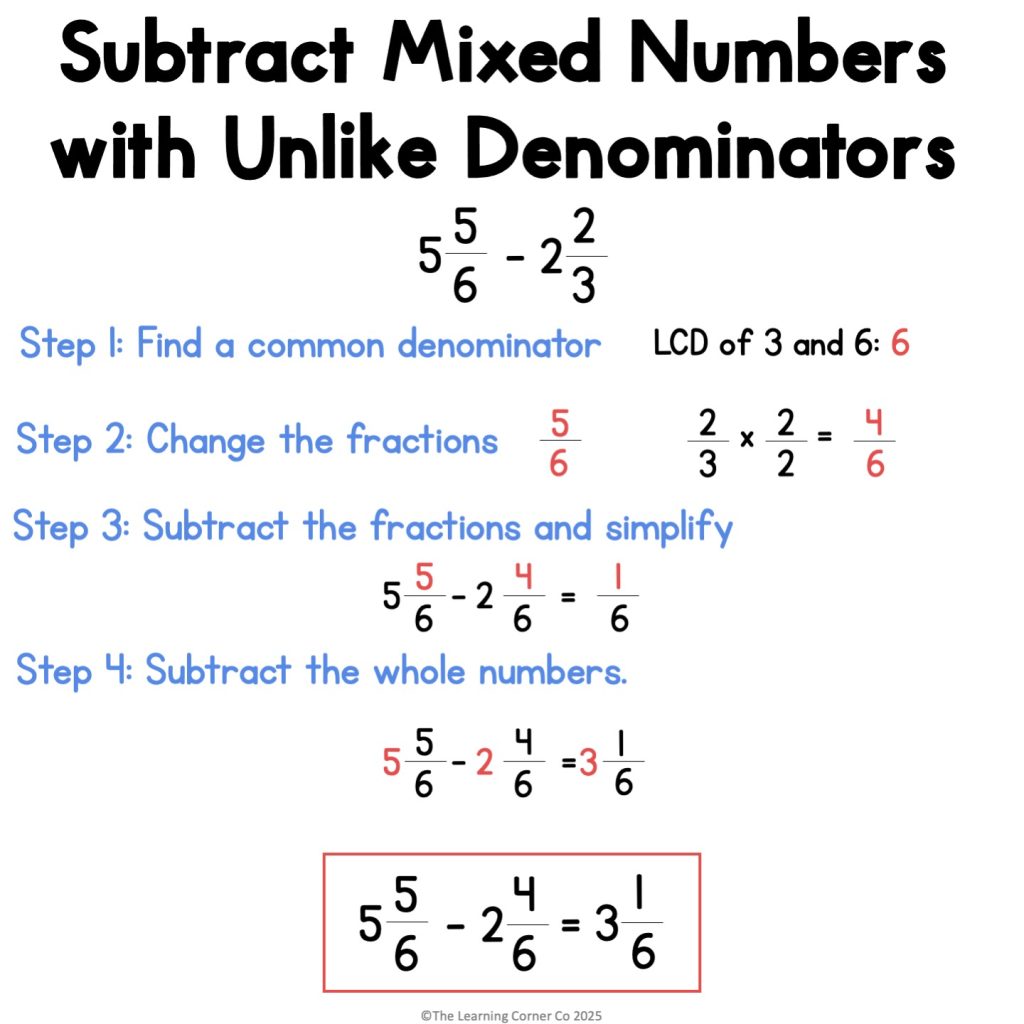
Example: Subtract 5 5/6 – 2 2/3
- Find the LCD of 6 and 3 → 6
- Rewrite:
- 5/6 stays the same
- 2/3 = 4/6
- Subtract the fractions: 5/6 – 4/6 = 1/6
- Subtract the whole numbers: 5 – 2 = 3
So, 5 ⅚ – 2 ⅔ = 3 ⅙
Subtracting Mixed Numbers with Borrowing (Renaming)
Sometimes when you find common denominators for the mixed numbers, the numerator of the first fraction is smaller than the second. When that happens, you need to borrow from the whole number before you can subtract.
Step 1: Find the Least Common Denominator
Look for a common multiple or multiply the denominators to find the LCD.
Step 2: Rewrite the Fractions
Change each fraction to have the same denominator.
Step 3: Borrow from the Whole Number
Take 1 from the whole number and turn it into a fraction that matches your denominator.
Step 4: Add the Borrowed Fraction
Add the borrowed fraction to the top fraction.
Step 5: Subtract Normally
Now you can subtract the fractions and the whole numbers like usual.
Example: Subtract 4 ¼ – 2 ⅝.
- Find the least common denominator of 4 and 8. → 8
- Rewrite the mixed number.
- 4 ¼ = 4 2/8
- 2 ⅝ stays the same
- Notice that 2/8 is smaller than ⅝.
- Borrow 1 whole from 4 and rename the whole as 8/8.
- Add 8/8 to 2/8 → 8/8 + 2/8 = 10/8.
- This means that 4 ¼ is also equal to 3 10/8.
Now, subtract the fractions, then the whole numbers.
- Subtract the fractions.
- 10/8 – ⅝ = 5/8
- Subtract the whole numbers.
- 3 – 2 = 1
So, 4 ¼ – 2 ⅝ = 1 5/8
Borrowing can feel tricky at first, and that’s okay!
If you want more step-by-step help, my Add and Subtract Fractions Workbook has a full section on subtracting mixed numbers, with lots of practice problems help your students master the skill.
👉 Take a peek at the workbook here!
Common Mistakes When Adding and Subtracting Mixed Numbers
Even when you know the steps, it’s easy to make small mistakes when working with mixed numbers. Here are some common errors to watch out for — and how to fix them!
1. Forgetting to Find a Common Denominator
When the fractions have different denominators, you must find a common denominator before adding or subtracting.
Example Mistake:
Add 2 ⅓ + 3 ¼
Mistakenly adding the fractions straight across: ⅓ + ¼ = 2/7 (incorrect)
Correct Way:
- Find the LCD of 3 and 4 → 12.
- Rename ⅓ and ¼.
- ⅓ = 4/12
- ¼ = 3/12
- Add the fractions.
- 4/12 + 3/12 = 7/12.
- Add the whole numbers.
- 2 + 3 = 5
So, 2 ⅓ + 3 ¼ = 5 7/12.
2. Not Borrowing When Subtracting
If the numerator of the first fraction is smaller than the second fraction when subtracting, you must borrow 1 from the whole number—forgetting to borrow leads to negative fractions or wrong answers.
Example Mistake:
Subtract 5 ⅙ – 2 ⅚
Trying to subtract straight across: ⅙ – ⅚ = -4/6
Correct Way:
- Borrow 1 from 5, making it 4.
- Add 6/6 to ⅙ → ⅙ + 6/6 = 7/6.
- Now subtract: 7/6 – ⅚ = 2/6 → simplify to ⅓
- 4 – 2 = 2
So, 5 ⅙ – 2 ⅚ = 2 ⅓.
3. Forgetting to Simplify the Final Answer
After adding or subtracting, always check if your answer can be simplified. A common mistake is leaving an improper fraction or a fraction that can be reduced to its simplest form.
Example Mistake:
Add 5 ⅛ + 3 ⅜ = 8 4/8
Error: Not simplifying the fraction 4/8
Correct Way:
- 4/8 simplifies to 1/2.
So, 5 ⅛ + 3 ⅜ = 8 ½.
4. Mixing Up Whole Numbers and Fractions
Some students accidentally add fractions to whole numbers or subtract whole numbers from fractions — mixing up the steps.
Example Mistake:
Add 2 ⅖ + 3 ⅗
Adding the whole numbers and the numerators incorrectly: 2 + 2 + 3 + 3 = 10
Correct Way:
- Add whole numbers: 2 + 3 = 5
- Add fractions: ⅖ + ⅗ = 5/5 = 1
- Then add: 5 + 1 = 6
So, 2 ⅖ + 3 ⅗ = 6
Quick Reminder:
Adding and subtracting mixed numbers gets easier the more you practice. Keep an eye out for these common mistakes, double-check your work, and you’ll get it right!
Need Extra Help with Adding and Subtracting Mixed Numbers?
✅ Looking for extra practice?
Check out my Adding and Subtracting Mixed Numbers Worksheets — they’re full of step-by-step examples, practice problems, and plenty of space to show your work. Perfect for building confidence one page at a time!
👉 Click here to grab the worksheets!
📘 Want even more support?
My Add and Subtract Fractions Workbook is packed with lessons, review pages, and extra practice to help you master adding and subtracting mixed numbers and fractions. It’s a great way to learn at your own pace and stay ahead.